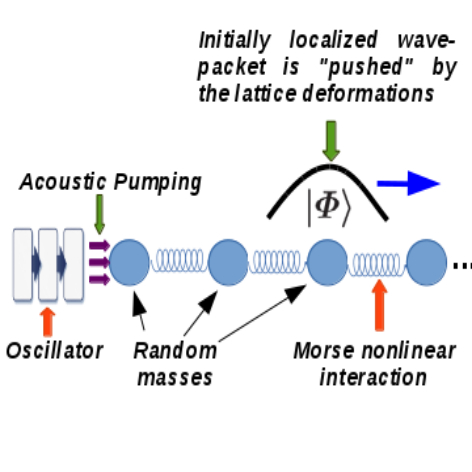
In this work we have investigated the dynamics of a single electron in a nonlinear chain subjected to disorder and in the presence of an external acoustic wave. We have used a quantum mechanics formalism to describe the electron transport and a classical nonlinear Hamiltonian for the lattice vibrations. We emphasize that our model contains two distinct sources of disorder: disorder within the on-site energies and at the mass distribution. Therefore, both dynamics quantum and classical equations contains disordered terms. The electron-lattice interaction was incorporated into the model through a hopping term defined as an exponential function of the effective distance between the nearest neighbors masses. By initializing the system with a fully localized wave packet at the left end of the chain and placing a Gaussian acoustic pulse generator at the same side, we observed that the acoustic wave associated with the electron-lattice interaction is able to sustain the wave packets dynamics even at the presence of intrinsic disorder (figure below by M.O.Sales). paper.

We consider interacting electrons moving in a nonlinear Morse lattice. We set the initial conditions as follows: electrons were initially localized at the center of the chain and a solitonic deformation was produced by an impulse excitation on the center of chain. By solving quantum and classical equations for this system numerically, we found that a fraction of electronic wave-function was trapped by the solitonic excitation and trapping specificities depend on the degree of interaction among electrons. Also, there is evidence that the effective electron velocity depends on Coulomb interaction and electron-phonon coupling in a nontrivial way. This association is explained in detail along this work. In addition, we briefly discuss the dependence of our results with the type of initial condition we choose for the electrons and lattice.To see more read the paper.

Considering non-interacting electrons in a one-dimension alloy in which atoms are coupled by a Morse potential, we study the system dynamics in the presence of a static electric field. Calculations are performed assuming a quantum mechanics treatment for the electronic transport and a classical Hamiltonian model for the lattice vibrations. Next, we report numerical evidence of the existence of a soliton-electron pair even when the electric field is turned on and we offer a description of how the existence of such phase depends on the magnitude of the electric field and the electron-phonon interaction. To see more read the paper.

We consider a simple 1d one-electron model containing the static randomness arising from the alloy composition and the coupling between electron and atomic vibration. The electron-vibration coupling used can be understood intuitively. The transfer integral between neighbouring atoms-also called the hopping term-represents the electron's kinetic energy and depends on the effective distance between neighbouring atoms. Basically, the hopping term increases when the distance between neighbouring atoms decreases. By using this formalism we have found a breakdown of Anderson localization. Our main result indicates that the coupling between atomic vibration and the electron's kinetic energy can promote a long-time subdiffusive regime. The numerical experiments were done by directly measuring the mean width of the electron wave function. To see more read the paper or the labtalk written to journal.

The absence of electronic self-trapping in one-dimensional lattices with nonadiabatic electron-phonon interaction. In ref. PRL we show that a delayed thirdorder nonlinearity destroy the usual delocalized-self-trapped transition present in nonlinear chains. We found that for the case of long delay times, self-trapping takes place only for much stronger nonlinearities. As a consequence, the electronic transport retains its metallic character in slowly responding media for a wide range of nonlinear strengths.

The effect of the on-site Hubbard interaction U on the dynamics of two electrons subjected to an external electric field and restricted to move in a linear chain with open boundaries. For electrons initially far apart, the wave-packet develops Bloch oscillations whose characteristic frequency is in agreement with a semi-classical calculation. For initially close electrons in a singlet state, a frequency doubling sets up, which is more pronounced for intermediate couplings. We discuss this effect by revealing the opposite trends the electron-electron coupling produces on the wave-packet components corresponding to bounded and unbounded states.

The existence of Bloch oscilations in low-dimensional systems with long-range correlated diagonal disorder. In ref. PRL2 we show that this type of correlated disorder does not destroy the coherence of Bloch oscilations.

The existence of extended states in low-dimensional disordered systems. In ref. PRL1 we found that the one-dimensional Anderson model with long-range correlated diagonal disorder displays a phase of extended electronic states. This theoretical prediction was experimentally realized in Germany by U. Kuhl and co-workers (see Appl. Phys. Lett. 77 633 (2000)). They examined the microwave transmission spectra of single-mode waveguides with inserted long-range correlated scatters. In good agreements with our theoretical calculations the experimental data demonstrated the existence of a transparent band due to correlations in disorder